Exercise 9.16 An harmonic oscillator?

Given is the circuit below; this circuit is intended to be an harmonic oscillator.

• The opamp is ideal, with a real valued voltage gain $A\to \infty$ and with no frequency dependency.
• The voltage buffer has a finite voltage gain $A_v$.
• The value of all resistors is the same ($R$); all capacitors have identical values ($C$).

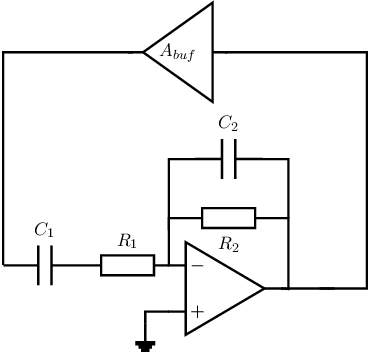
(a)

Which condition(s) must be satisfied to get harmonic oscillation?

(b)

Determine whether the given circuit can function as harmonic oscillator using a positive, real valued $A_{\mathit{buf}}$. If your answer shows that harmonic oscillation can be possible, derive an expression for the (radian) oscillation frequency and for the required $A_{\mathit{buf}}$. Otherwise demonstrate clearly (mathematically) that this circuit cannot oscillate harmonically.

(c)

Determine whether the given circuit can function as harmonic oscillator using a negative $A_{\mathit{buf}}$. If your answer shows that harmonic oscillation can be possible, derive an expression for the (radian) oscillation frequency and for the required $A_{\mathit{buf}}$. Otherwise demonstrate clearly (mathematically) that this circuit cannot oscillate harmonically.

(d)

In the previous question, a real valued $A_{\mathit{buf}}$ was assumed. For (one of) the case(s) that the circuit oscillates harmonically, show whether the oscillation frequency increases or decreases is the buffer has a (small) negative phase shift (due to finite bandwidth). Showing this can be done by a mathematical treaty, graphics constructions, ... but needs to exceed guessing.