Exercise 9.14 An harmonic oscillator?

Given is the circuit below; this circuit is intended to be an harmonic oscillator.

• The opamps are ideal, with a real valued voltage gain $A\to \infty$ and with no frequency dependency.
• The value of all resistors is the same ($R$), except for the rightmost resistor (that one has value $R_1$). Whenever applicable, all capacitors have identical values ($C$), and all inductors have identical values ($L$).

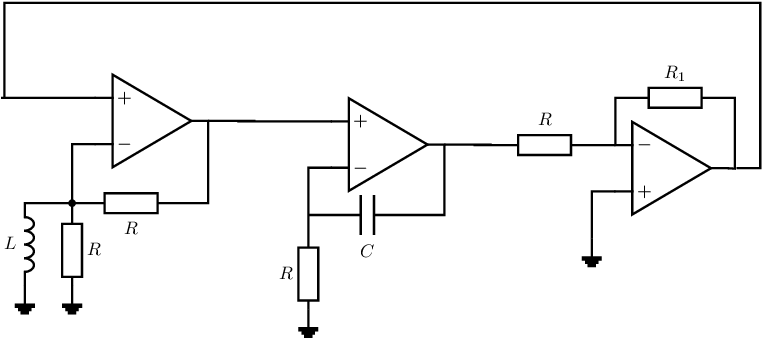
(a)

Which condition(s) must be satisfied to get harmonic oscillation?

(b)

The circuit can be decomposed into 3 stages. Each stage starts at the output node of the previous opamp and ends at the output node of the opamp in the stage.
Derive the voltage transfer for each of the 3 stages.

(c)

What range of phase shift can be achieved by the three stages, for $0\le \omega \le \infty$? Note that your answer consists of three phase ranges, one per stage.

(d)

Determine whether the given circuit can function as harmonic oscillator. If your answer shows that harmonic oscillation can be possible, derive an expression for the (radian) oscillation frequency and for the required $R_1$. Otherwise demonstrate clearly (mathematically) that this circuit cannot oscillate harmonically.

A similar but different circuit schematic is shown below. Also this circuit is intended to be an harmonic oscillator.

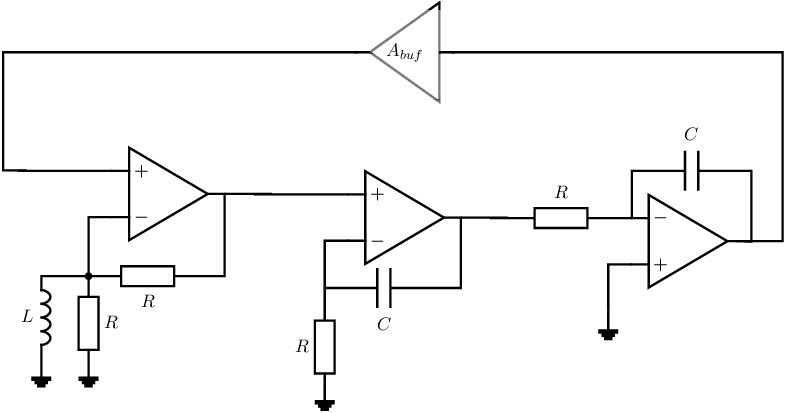
(e)

Also this circuit can be decomposed into 3 stages (and an extra voltage gain stage having a real valued voltage gain $A_{\mathit{buf}}$). Each stage starts at the output node of the previous opamp and ends at the output node of the opamp in the stage.
Derive the voltage transfer for each of the 3 stages.

(f)

What range of phase shift can be achieved by the three stages, for $0\le \omega \le \infty$? Note that your answer consists of three phase ranges, one per stage.

(g)

Determine whether the given circuit can function as harmonic oscillator, assuming a real valued $A_{\mathit{buf}}$. If your answer shows that harmonic oscillation can be possible, derive an expression for the (radian) oscillation frequency and for the required $A_{\mathit{buf}}$. Otherwise demonstrate clearly (mathematically) that this circuit cannot oscillate harmonically.