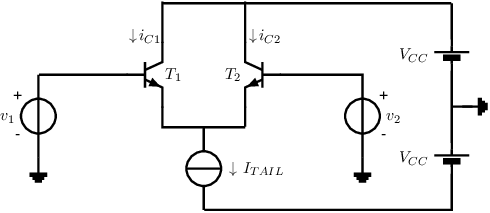
Exercise 8.1 A differential pair

Given is a differential pair with NPNs as shown below. In this circuit, transistors $T_1$ and $T_2$ are identical with $\beta fe=250$. As a good approximation, the collector current as a function of base-emitter voltage is according to:

The current source $I_{TAIL}$ can be considered ideal.
For room temperature $\frac{kT}{q}=25mV$ and for this circuit $I_{TAIL}=1mA$.

a)

Derive expressions for $i_{C1}$ and $i_{C2}$ as a function of ($v_1$ – $v_2$).

b)

Sketch the relation between $i_{C1}$ and ($v_1$ – $v_2$) for $-\frac{4kT}{q}<$ ($v_1$ – $v_2$)$<$ $\frac{4kT}{q}$.

c)

Derive an expression for the transconductance $gm$ of $T_1$ and $T_2$ for $v_{IN}=v_1-v_2=0$ and calculate the numerical value.

d)

What could be an advantage of making $I_{TAIL}$ proportional to the (absolute) temperature?

e)

If the current source is not ideal but has an output resistance of $100k\mathrm{\Omega }$, calculate the percentage change in $g_m$ for a common signal with a value of $2V_{peak-peak}$.

Next, the input stage is used in an amplifier with feedback. In this case, $v_1=v_{in}$, $v_2=\beta \cdot v_{out}$, $v_{out}=A(v_1$–$v_2)$, $A=100$.

f)

Derive an expression for the maximum amplitude of the input voltage $v_{in}$, so that the differential input voltage will not be larger than a pre-specified $v_{diff,max}$. Calculate the numerical value of this amplitude if $v_{diff,max}=0.4\frac{kT}{q}$ and $\beta =0.1$.